Crouching Stepper, Hidden Rider
This variant was inspired by, of all things, the same image being widely used for the long-range Cannon and the relatively short-range Dabbaba. These together with the Rook and Wazir are the four historic symmetric radial pieces, and all have diagonal counterparts of which only the Arrow is a recent innovation. Of the resulting octet of piece types, Xiang Qi uses two aside each of four - one type in each range and two types in each kind of direction. There is also a single royal piece that moves like a Wazir.Ways have been considered of involving the moves of all eight types among the capturable pieces. One is combining each piece with its dual, either absolutely as in my own Alibaba Qi or limited to different directions from different cells as in Fergus Duniho's Storm the Ivory Tower. Another approach is to have pieces of fixed direction switching from long-range to short-range and back according to which cells they are on. This would be in accord with the Cannon/Dabbaba image sharing. It was just a matter of finding the right way to divide up the board.
I decided to have large blocks of cells with common rules, to simplify the concept compared with the Ivory Tower. Dividing up the files was obviuos enough: as 9 is 3 squared I could do little but divide into 3 3-file groups. For dividing the ranks, 10 is 5x2, giving the choices. Using the traditional River to have 2 5-rank groups gave 6 15-cell blocks, which I felt too large and few. It also put 3 of the relevant piece types into the same kind of block, which I judged asymmetric. I therefore decided to have 5 2-rank groups and so 15 6-cell blocks.
The name is of course inspired by the film Crouching Tiger, Hidden Dragon, because the transformation from short-range to long-range suggests a martial artist uncurling from a crouching position.
Setup
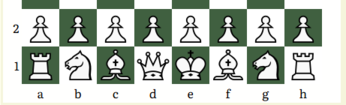
As Xiang Qi except that instead of being divided in two by a River (although there is a river Crouch!) the board is divided into 15 by 4 rank boundaries and 2 file boundaries, with the Fortress occupying each General's block and all rather than half the one in front. For ease of reference I label the 4 corner blocks and the 4 blocks bordering the central one Yellow, and the central block and the 6 blocks bordering the corner ones Purple. These are two opposite colours not used in Xiang Qi itself. A standard Xiang Qi set may be used as piece changes are determined entirely by position, making Shogi-style flipping unnecessary.
Pieces
Each capturable piece moves differently depending on what colour block it is in. With the exception of those directly between the Generals, all start off in blocks where they have the move of the corresponding Xiang Qi piece.The corner pieces move like ROOKS from cells in Yellow blocks (including where they start), and like WAZIRS but not across Fortress boundaries from cells in Purple blocks. A move of 2 or more steps into a Purple block is not reversible.
The next pieces in move like MAOS from cells in Yellow blocks (including where they start), and like MOAS from cells in Purple blocks. These are not part of the general radial pattern, but it seemed logical to give them varying moves as well. Moves switching block colour are reversible for this piece.
The next pieces in move like stepping ELEPHANTS from cells in Yellow blocks (including where they start), and like ARROWS from cells in Purple blocks. They cannot move through an intervening piece when Elephants or when not capturing, but cannot capture without such a piece when Arrows. A move of 3 or more steps (or a noncapturing move of a single step) into a Yellow block is not reversible. Colourbinding allows one Elephant/Arrow to cover the other of its army, but not capture an enemy one.
The pieces flanking the General move like BISHOPS from cells in Yellow blocks and FERZES but not across Fortress boundaries from cells in Purple blocks. This means that they must move as in Xiang Qi until they reach their third rank. A move of 2 or more steps into a Purple block is not reversible. Colourbinding allows one Bishop/Ferz to cover the other of its army, but not capture an enemy one.
Colourbinding also allows a Bishop/Ferz to capture an enemy Elephant/Arrow, and vice versa, but one of each of the same army cannot cover each other. Note that short-range pieces are associated with long-range ones the opposite way to that of Fergus Duniho's Yang Qi. This is to match the Orthogonal pairings.
The pieces on their players' third ranks move like stepping versions of DABBABAS from cells in Yellow blocks, and like CANNONS from cells in Purple blocks (including where they start). They cannot move through an intervening piece when Dabbabas or when not capturing, but cannot capture without such a piece when Cannons. A move of 3 or more steps (or a noncapturing move of a single step) into a Yellow block is not reversible.
The pieces on their players' fourth ranks follow slightly more complicated rules. They always move exactly one step orthogonally, but it must be backward from the enemy General's block, forward from other Purple blocks, and left or right from Yellow blocks. This allows them to circulate endlessly on enemy ground until captured.
The GENERAL is unchanged from Xiang Qi except that it is confined to a larger Fortress.
Rules
As in Xiang Qi there is no initial double-step move, En Passant, or Castling.There is also no promotion in the sense that crossing the River in Xiang Qi can be seen as promotion.
As in Xiang Qi, victory is by Checkmating the enemy General, and Generals are not permitted to share a file without an intervening piece.
This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
By Charles Gilman.
Last revised by Fergus Duniho.
Web page created: 2006-03-27. Web page last updated: 2022-12-04